Relationships Regarding Select Physical and Chemical Constants

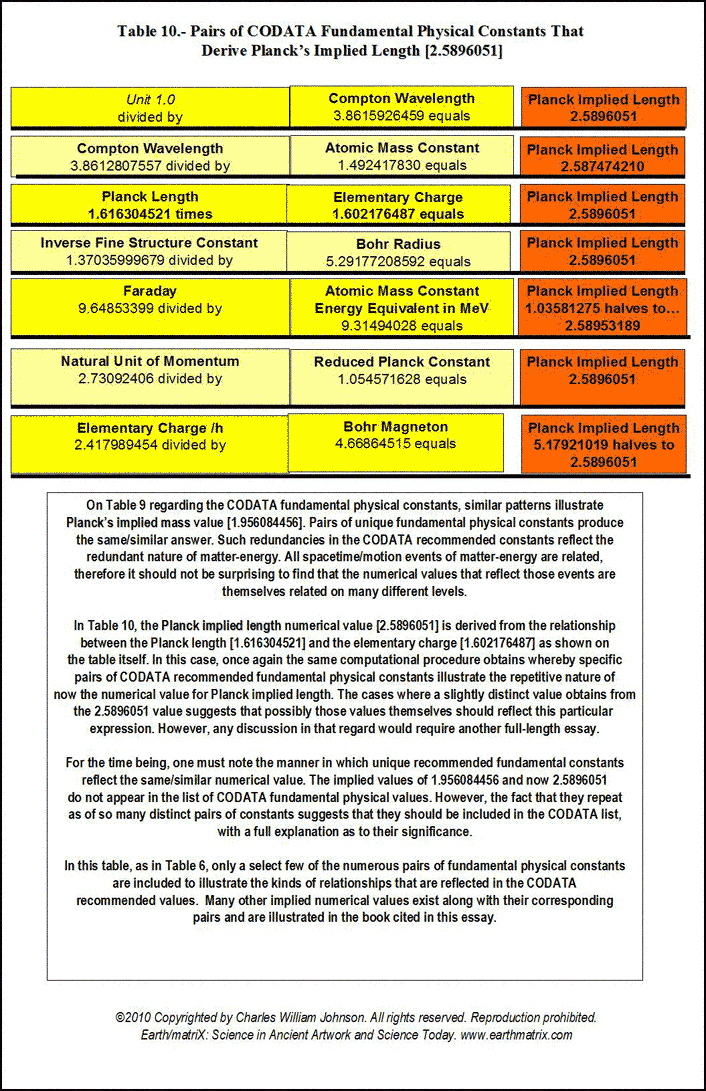
In this essay, interrelationships of selected fundamental physical and chemical constants are examined as of the CODATA recommended values, as well as of the implied Earth/matriX values. The propositons regarding the analysis of interrelationships of select physical and chemical constants is presented in the order in which they appear in one of my notebooks. Numerical values are presented in their fractal expression with a floating decimal place. Numerical values vary according to certain computational procedures, but in general the first five significant digits determine the relevancy of the computation.

1.- The von Klitzing constant is a derivative of a 1.366c numerical value. For an extended list of physical and chemical constants reflecting a 1.366c numerical value, visit www.earthmatrix.com.

4.- The natural unit of energy in MeV is the reciprocal of the Planck implied energy.

5.- Inverse fine structure constant divided by Bohr radius yields Planck implied length.

7.- The reciprocal of the atomic unit of permittivity is the square value of the speed of light in vacuo.

10.- The reciprocal of the magnetic flux quantum is the conventional value of the Josephson constant.

11.- Half of the fractal numerical value of the conventional value of the Josephson constant is the elementary charge as e/h.

12.- The elementary charge divided by Planck's constant is the elementary charge as e/h.

13.- The reciprocal of the magnetic flux quantum divided by the elementary charge yields fractal inverse of conductance quantum.

14.- Revised computation. The reciprocal of Planck time yields a distinct value for the atomic unit of magnetic dipole moment, which in turn yields a distinct value for the Bohr magneton. In this sense the derivative of Planck time contradicts the numerical value for the Bohr magneton.

15.- The revised Bohr magneton value divided by the elementary charge yields a distinct fractal value for the Bohr magneton in eV T-1.

16.- The elementary charge divided by the conventional value of the Josephson constant yields half the value of the Planck constant.

17.- The conventional value of the Josephson constant divided by the inverse conductance quantum yields a near fractal value of the speed of light in vacuo. Variations in the value call for theoretical consideration of terms.

18.- There are about thirty pairs of fundamental physical constants in the CODATA that produce the fractal numerical value of the elementary charge. Here are a few relevant examples of many. The magnetic flux quantum times the conductance quantum yields the elementary charge.

The magnetic flux quantum divided by the elementary charge yields the inverse conductance quantum.

The conductance quantum divided by the elementary charge yields the conventional value of the Josephson constant.

With the previous three examples, one should begin to get the feel for how the recommended fundamental physical constants are interrelated as of their fractal numerical values. For even though each constant, with its own proper name, appears to be self-sustaining and significant, actually obtains its meaning from the interrelationship with the other fundamental physical constants.

19.- This proposition should have headed the list presented here, but the purpose is to follow the flow of the notebook and how the numbers derived thereof. The elementary charge is a near fractal multiple expression of Planck's constant. From this perspective, one may understand how so many interrelationships appear regarding the elementary charge as a function of Planck's constant, or vice versa.

20.- The electron charge to mass quotient divided by pi yields a fractal multiple expression of the Bohr magneton in eV t-1 / h.

21.- The proton-electron mass ratio divided by the Planck implied energy yields a near multiple expression of the proton mass energy equivalent in MeV [938.272013].

The proton mass energy equivalent in MeV divided by the Planck implied energy value yields a near fractal multiple of the speed of light in vacuo.

22.- The neutron-electron mass ratio divided by the neutron mass energy equivalent in MeV yields a near fractal multiple of Planck's implied energy. The order in this selection is inverted simply for the sake of flexibility in the analysis of the interrelationships. In other words, there is no specific order to be followed in discerning the interrelationships.

The significant point in the analysis is to recognize the meaning of the Planck implied values and their need to be taken into consideration in the CODATA listing of recommended values. In other words, the Planck implied values are not merely computational in nature but represent constant relationships within spacetime/motion and the specific expressions of matter-energy.

23.- The elementary charge divided by the Planck implied energy yields an inverse fractal expression of Planck energy, whose reciprocal is Planck energy of course.

The inverse Planck energy, 8.19073267, doubles to 6.552586136, reflective of Planck's second choice for the value of his constant [6.55] forwarded by him in 1900.

24.- Planck mass times Planck implied energy yields near fractal multiple of the [shielded] proton gyromagnetic ratio /pi [4.25774821; 4.25763881].

25.- The [shielded] proton gyromagnetic ratio divided by the Planck implied energy yields a near fractal multiple of the Newtonian constant of gravitation [6.67428].

One cannot help but notice the particular relevancy of the Fermi coupling constant [2.2255] to the Newtonian constant of gravitation:

26.- The reciprocal of the square of the speed of light in vacuo yields a near fractal multiple of the Fermi coupling constant [2.2255].

27.- Interrelationships for Planck implied length are presented in the following table.

Once again, the examples presented on the following table that illustrate how specific pairs of fundamental physical constants derive the Planck implied length are only a few of many.

Such are the relationships of redundancy among the CODATA recommended fundamental physical constants that numerous tables would be needed to illustrate each particular implied fractal value. In various essays and in one of my recent books about the Planck constants, I have presented numerous examples of the Planck implied values that do not appear in the CODATA listing. In my view, these particular relationships and implied fractal values need to be incorporated into the CODATA listing along with a detailed theoretical explanation as to their derivation and meaning with regard to all of the other fundamental physical constants.

28.- The fine structure constant represents a near fractal reciprocal multiple of the elementary charge [1.602176487].

29.- The natural unit of time multiplied by the Planck implied time yields a near fractal multiple of the atomic unit of permittivity [1.11265056].

Check my previous essay and book on Planck implied values, as in Planck time 5.39124 times the elementary charge, 1.602176487, equals the Planck implied time: 8.637717964.

With the previous list, it should be apparent by now that the Planck implied values are not randomly coincidental, but reflect relationships of spacetime/motion in specific forms of matter-energy. From this list, it also becomes evident that one may not only base the derivation of the Planck constants on the fundamental physical constants, but do likewise regarding many of the other recommended CODATA values.

Note that the many diverse interrelationships of the fundamental physical constants and the Planck constants are carried out with basic math. Given the fact that all of spacetime/motion is relational, and that all forms of matter-energy are relational, it is possible to derive all of the fundamental physical constants in the manner illustrated in this brief selective summary. At least, this is the case when the fundamental physical constants reflect exact and precise measurements.

THE PLANCK CONSTANTS BASED ON THE FUNDAMENTAL PHYSICAL CONSTANTS AND ERRORS AND OMISSIONS OF THE CODATA

The author derives the Planck constants as of the fundamental physical constants, instead of as of the traditional symbolic formulae given in the CODATA. The implied numerical values that are generally omitted in the science literature and which serve as a basis for deriving the Planck constants are identified in detail in their basic math. As of the analysis of the implied Planck values, errors and omissions of the CODATA Planck values are identified and treated extensively. The Planck constants are reverse engineered in order to employ the fundamental physical constants as their computational foundation. Various Earth/matriX Tables of the Planck Constants Based on the Fundamental Physical Constants are presented for the first time, illustrating how the fundamental physical constants serve as the foundation to the Planck constants.

Purchase and download the complete e-book:
Errors and Omissions in the CODATA and The Planck Constants Based on the Fundamental Physical Constants
PDF file 142 Pages
Earth/matriX Editions ISBN 1-58616-463-5
Price: $8.19US Introductory Offer