AND
SCIENCE TODAY
Extract 49
The Anti-Radian: 5.28318530751
by Charles William Johnson
The diameter of a circle may theoretically be divided into its
circumference 3.141592654 times, or pi times, while, the radius,
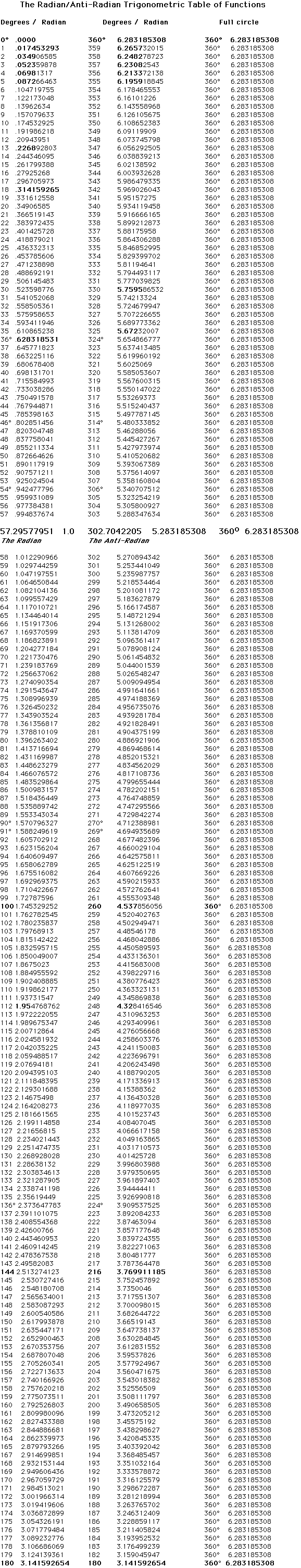
may theoretically divide into the circumference 2![]() (6.283185308) times. The length of the radius marked off on the circumference
of the same circle is called the radian (57.29577951).
There is no name for the length of the diameter marked off on
the circumference of the circle as far as we know. Two radians would represent
the distance of the diameter (114.591559). The history of mathematics
has it, however, that the radian is significant in this sense, while the
length of the diameter remains significant moreover in relation to the
concept of pi (diameter: circumference).
(6.283185308) times. The length of the radius marked off on the circumference
of the same circle is called the radian (57.29577951).
There is no name for the length of the diameter marked off on
the circumference of the circle as far as we know. Two radians would represent
the distance of the diameter (114.591559). The history of mathematics
has it, however, that the radian is significant in this sense, while the
length of the diameter remains significant moreover in relation to the
concept of pi (diameter: circumference).
The radian represents essentially a sector of the circle, marking off 57.29577951
degrees of a 360-degree circle. The number 360 has been historically established
and conventionally accepted as representing the number of theoretical sectors/degrees
within a circle. Obviously, a circle may have as many sectors/degrees as one
might wish to physically divide the circle into for computational reasons. The
ancients appear to have divided a circle into any number of sectors/degrees.
The numbers cited above remain as constants to any relationship of the circle
in terms of its diameter/radius and circumference. The number of sectors/degrees,
however, shall vary in their symbolic representation, whether it be a circle
of 360 degrees or 260 sectors (if we do not like to use the word "degrees"
in this latter case).
Contemporary electronic calculators are engineered to function as of the historically
accepted 360-degree circle. In order to translate angles from a 360-degree circle
to a 378-sector/degree circle, one would have to employ another constant (1.05)
as of these numbers 378/360 = 1.05). The concept of the radian is also generally
offered in relation to the specific 90-degree angle of circles. In examining
al most any table in any textbook on mathematics about Trigonometric Functions
of Angles in Degrees, it becomes obvious that information is offered regarding
angles within the 90-degree boundary. For example, on one side is listed the
degrees of angles in radians for 0 to 45 degrees, and on the opposite side of
the table appear the degrees of angles in radians for 90 to 45 degrees. Such
a procedure can be confusing in selecting opposites which are simply complementary
angles. To compare a complementary angle of 30 degrees with its complementary
angle of 60 degrees has nothing to do with the concept of the radian. One has
merely compared part of a radian to another radian. The comparison that is required
for us to better understand the concept of the radian is to compare the radian
to the remainder of the angle in the circle, that is, to the anti-radian.
Given that the radian, the minor sector, represents 57.29577951 degrees of the
360 degrees in a circle, then subtracting the radian from the circle means that
the remainder of the circle, the major sector, that is, the anti-radian,
represents 302.7042205 degrees.
The circumference of the circle contains 2 radians. If we subtract one radian from that figure, we then obtain the number of radians in the circumference of the anti-radian.
The anti-radian represents 5.283185308 radians, or 302.7042205 degrees of the circle. It would appear as though the ancients may have conceived of the radian in opposition to the anti-radian (as of the 360 degrees of a circle), as we have explained in previous extracts (Nos. 47 & 48). Contemporary textbooks on mathematics conceive of these relationships of angles in a different manner as of the right angle (90 degrees). These different conceptions may explain in part some of the ancient designs.
For example, in a contemporary textbook, on the tables of Trigonometric Functions of Angles in Degrees, one may observe the opposition of angles of 36 degrees and 54 degrees. Thirty-six degrees represents, according to the textbook, .6283 of a radian (i.e., 35.99893827), whereas fifty-four degrees represents .9425 of a radian (i.e., 54.00127219). And, obviously, the sum of these two angles is that of ninety degrees. However, a distinct symbolism is acquired by making the comparison to the total number of degrees/radians in a circle, and not simply to ninety degrees (one-fourth of a circle).
Let us review these numbers in terms of the circle.
Now, let us subtract this figure from the total radians in a circle (2![]() ):
):
Now, for the fifty-four degree example.
By thinking radian/anti-radian, the comparison of the numbers in degrees and percentages, are more relational. Whereas, by thinking in terms of a right angle, one has to relate the percentages of a radian to that of ninety degrees (.1.5708).
| 36° .6283 | [90° 1.5708] | .9425 54° |
In the textbook, the implied comparison between a 36 degree angle and a complementary 54 degree angle is made in relation to the ninety degree angle. However, the percentages offered for each one are in relation to the radian of 57.29577951 degrees which in turn is in relation to the full circle of 360 degrees.
Obviously, then, for the sake of comparison and a full theoretical understanding of the relationship between mathematics and geometry, the tables of trigonometric functions should be better served were they offered directly in relation to the 2-pi relation (6.283185308) of the full 360-degree circle, as follows.
| 36° .6283 | 324° 5.654866777 | 360° 6.283185308 |
| 54° .9425 | 306° 5.340707512 | 360° 6.283185308 |
| 90° 1.5708 | 270° 4.712388981 | 360° 6.283185308 |
In this manner, with one single table of trigonometric function, one may better observe the comparisons between complementary, supplementary angles, as well as in relationship to the entire 360 degrees of the circle.
The ancients may have conceived of the significance of the anti-radian in such a comparison. For example, let us examine some of the previous angles to the anti-radian.
| 302.7042205 / 324 degrees = | .934272285 |
| 1.868544571 | |
| 302.7042205 / 306 degrees = | .989229479 |
| 1.978458958 |
Now, consider a little reverse engineering of numbers. An historically significant number for the maya long count system is that of 1872000. Consider it as a fractal expression and reverse the computation as follows:
| .936000 | ||
| 302.7042205 / 1.872000 | = | 161.7009725 |
| 323.401945 | ||
| 360 - 323.401945 | = | 36.59805502 |
From this computation, we may observe a direct relationship with an angle that could possibly represent the 365c fractal day-count in relation to the maya long count period number (1872000).
Now consider, the 54 degree angle computation, which is suggestive of the Nineveh number/fractal 1959552.
| .979776 | |||
| 302.7042205 / 1.959552 | = | 154.4762377 | |
| 308.9524754 | |||
| 360 - 308.9524754 | = | 51.0475246 | [a figurative suggestive of some of the angles of inclination of the pyramids in ancient kemi] |
Adjustments could be made to where one might visualize an encoding of numbers/fractals related to the purely mathematical aspects of geometry, as well as to the symbolic numbers/fractals representing events in the cosmos. If the ancients were measuring the radian, they were more than likely also measuring the anti-radian. This they were most undoubtedly achieving in relation to the entire 360-degree circle, and not simply in relation to right angles.
For example, in ancient Mesoamerica calendrical counts are based on the 260c and 360c day-counts. Interestingly enough, 260 degrees on a 360 degree circle represents 4.537856056 radians (130:180 degrees ---2.268928028 radians). One can imagine the ancients rounding off to the historically significant fractal/number of Nineveh which is 2268 and its double, which is 4536. That would represent a very close approximation. We have already seen how the 2.268 fractal/number is in a pi-like relationship to a 260-degree/sector circle in previous essays and extracts. It just may be that the relationship between the 260c and the 360c has more pure mathematical bases than formerly suspected. Once again, we may observe a relationship with pure math, and in this case it has to do with the concept of radians.
***
The Anti-Radian: 5.28318530751
Extract 49
Earth/matriX: Science in Ancient Artwork,
25 April 1999.
e-mail: johnson@earthmatrix.com
Earth/matriX:
P.O. Box 231126,
New Orleans LA 70183-1126.
USA
***
Earth/matriX: Science in Ancient Artwork,
Your comments and suggestions are greatly appreciated:
e-mail: johnson@earthmatrix.com
| Home | Books | Forum | Reviews | Links | Author |