E a r t h / m a t r i X
SCIENCE IN ANCIENT ARTWORK
AND
SCIENCE TODAY SERIES
Essay N°120
The Radian and the Anti-Radian:
A Method of Reckoning
by Charles William Johnson
Chapter II
One of the reasons that scholars may have not been able to discern the method of reckoning behind the ancient systems has to do with the manner in which we have learned our mathematics and geometry. These subjects are generally taught in abstracted terms through general rules and formulae. The actual numbers of specific cases are arbitrarily chosen as examples to illustrate those rules and exercise the formulae. The numbers in the cases are never really written out in a systematic manner. In other words, the established teaching methods, tried and true, do not readily allow us to see how the numbers behave and perform in a systematic manner.
Throughout the Earth/matriX series of essays and extracts, we have been listing tables and tables of numbers that would appear to be repetitious and probably causing impatience in some readers. That is part of our way of learning; we wish to get to the bare, essential point immediately. However, by listing the numbers in a systematic manner, we are able to obtain a better feel for the numbers themselves and thereby notice the patterns being established. The ancients appear to have discerned these patterns, and it is these patterns which allow for a possible comprehension of their method.
In teaching students about the concept of pi, generally a brief explanation is offered along with the current day figure used to reflect pi, the 3.1416 or 3.141592654 figures. Upon studying the radian, students simply learn the figure 57.29577951 or 57.3, as representing the radian, and possibly the reciprocal number of .017453293 or .01745, without even discussing the complete circle. To conceive of the radian, one must necessarily comprehend the concept of the anti-radian, that is, the remaining sector of a circle outside the radian, whose figure is 302.7042205 degrees/units, and which together with the radian make up the full circle of 360 degrees. In fact, it has been our drawing attention to the anti-radian which has allowed us to visualize the complete concept employed by the ancients. It is impossible (although we do it) to conceive of the radian without thinking about the circle. Yet, we generally ignore and discard the sector concerning the anti-radian in our computations without any problem or difficulties arising thereof.
Nevertheless, the ancients may have visualized the radian and the anti-radian as they visualized the complete circle, that is, as the circle actually exists in its entirety. By comprehending the relationship of the radian and the anti-radian, one is then able to comprehend the relationship of the distinct day/year counts and cycle counts without much trouble. Without the full-circle concept, those counts may simply be conceived in isolation from one another and remain unrelated. Most scholars do not recognize the ancients as having known of the concept of pi; much less the concept of the radian; and even much less the concept of the anti-radian. Any attempt to illustrate that the ancients knew of these relationships can only be met with disbelief. Yet, as we shall observe below, the numbers and fractals coming out of the different ancient reckoning systems directly reflect these features of mathematics and geometry. In fact, one may draw the conclusion that many of the day/year counts were chosen based on these theoretical abstractions, and not on empirical observation of the skies. The 260c day-count and the 360c day-count of the ancient reckoning systems have been thought to represent empirical choices based on erroneous conclusions about the length of the year. From the theoretical math and geometry, it would appear that these counts reflect a method of reckoning determined by the very logic of numbers coming out of the features of a circle.
The rotation of the planetary bodies suggests circular movements in the sky. To have chosen a mathematical system for reckoning those timings based on the theoretical functioning of a circle would surely be a product of scientific reasoning. The ancients chose the 260c and the 360c, not from empiricism of astronomical observation, but from theoretical study of abstracted mathematics and geometry. Essentially, that is what we shall illustrate in this essay in a manner that suggests that the ancient reckoning systems are based in scientific inquiry. And, in fact, the ancient system, although we may not know how to actually employ it today, from the following analysis, appears to represent a more dynamic and flexible method for reckoning time than our own fixed method based on a specific time frame: that of Earth's rotation.
In other words, the contemporary method is a case-specific method of reckoning, while the ancient method of reckoning appears to be more theoretically and abstractly oriented applicable to any case. Without wishing to qualify one method as being superior or inferior to the other, we shall simply state that the ancient method may apply to all cases, whereas the contemporary system applies to a singular case.
The Radian and Anti-Radian Table of Functions
In order to enter into our analysis, we must present once again the table of functions for the radian and the anti-radian. The radian represents the length of the radius of a circle marked off along that circle's circumference, which embraces 57.29577951 degrees/units of arc. The remaining sector of the circle, thus marked off by the radian, may be referred to as the anti-radian and embraces the remaining arc, which consists of 302.7042205 degrees/arc. The sum of the measurement of the radian and the anti-radian equals the 360 degrees/units of a circle as we know it. The 360c degrees on a circle is an historically established figure, whose reasons for being lie beyond the confines of this essay. The ancient reckoning system appears to have employed circles of different units/degrees (260c, 354c, 374.4c, 378c, etc.). Today's system is case specific, limited to only one particular case; that of 360c degrees. To even think of a circle as having more or less than 360 degrees is something not to be found in contemporary textbooks in general. By today's definition, then, a circle has 360c degrees. In our mind, the ancients appear to have visualized the circle as having as many degrees/sectors as possible or necessary, from one to infinite number (and possibly, without entering into a philosophical discussion, from none to infinite).
The ancients probably knew the concept of pi (3.141592654), the radian and the anti-radian. They probably knew that these relationships were constant for any circle, varying symbolically in number in accordance with the numbers of degrees/sectors in a circle. Furthermore, the ancients possibly had a concept or theoretical visualization, not only of the radian (the length of the radius on the circumference of the circle), but of the length of the diameter on the circle. We have no special word for this, that we know of; we just call it two radians (114.951559). One could think of the existence of a radian (the length of the radius on the arc of a circle, 57.2957795°), and its double, the diametian (the length of the diameter on the arc of the circle 114.951559°). The word diametian obviously does not exist, but we may use it simply for economy of reference.
Generally, textbooks offer the numbers related to degrees and their radians on a 360c degree circle for the 90-degree sector only. The numbers are offered of complementary angles only. Other tables, in an effort to be more complete, offer supplementary angles as well. However, to offer figures related to ninety-degree angles or 180-degree angles or less, does not reflect the complete information about a 360-degree circle. We have therefore offered the information with regard to the two sectors from 1 to 180 degrees, and from 180 to 360 degrees. In this manner, we have a complete picture of the radian numbers relating to the 360 degrees of a circle. Today's teaching has it such, that no word is generally offered in textbooks for these two different angles (other than one is minor and the other major). But, apparently, there is no complementary or supplementary word-concept for these kinds of related angles in opposition to one another. The radian is simply taught as being the radian, with no reference to the remaining sector of the circle.
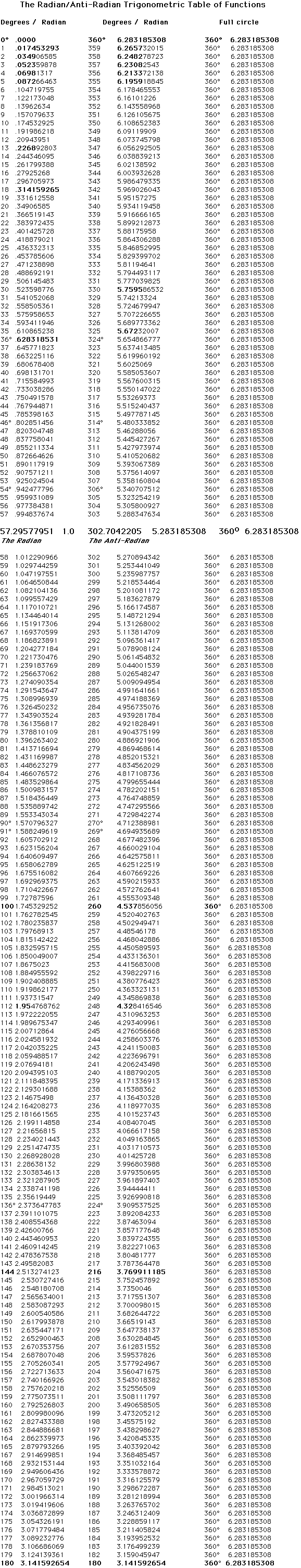
On the previous table, we have drawn attention to the placement of the radian and the anti-radian within the 360 degrees/units of the circle.


In teaching geometry and trigonometry, there exists a certain economy in saving space and effort. The trigonometric tables generally show only the range for the zero to ninety degrees of a circle, given that the remaining degrees may be derived from the 0-90 range. However, by not showing the entire gamut of numbers/degrees within the circle, it is almost impossible to discern what the ancients possibly already knew. If a circle is theoretically divided into two sectors, then knowing one sector immediately implies knowing the other. However, one should follow through on realizing the computations of each sector. To know the radian without exploring the anti-radian, for example, hides half of the problem and thereby half of the solution to any problem.
It has often been pointed out that the number of degrees in the radian (57.29577951 or 57.3) is suggestive of some ancient reckoning numbers of the maya system such as the fractal 576 number. But, the picture is completed, when we consider that the anti-radian portrays a number 302.7042205 that is suggestive of the fractal number 3024 (4 x 756) of the perimeter of the base of the Great Pyramid in Giza. We have explored this particular case in a previous extract (Cfr., Earth/matriX Extract No. 48). In earlier writings, we have alluded to the possibility of the ancient kemi as having employed a 378c circle, as well as the 360c circle. The ancient maya employed a 360c circle, and also possibly a 378c circle according to writers like Hugh H. Harleston, Jr. Now, consider the relationship of the numbers/fractals to these counts.
As the reader reviews the following analysis, one should keep in mind the use of a floating decimal point regarding the concept of fractal numbers. Therefore, consider the following possibility:
| 360 - 302.4 = 57.6 (maya) | 378 - 302.4 = 75.6 (kemi) |
To find such a coincidence in the computations, as we have been illustrating throughout our research findings, somehow defies logic. To argue that the ancient reckoning systems and the historically significant numbers/fractals are related due to chance, seems an untenable position. Yet, many scholars are not willing to consider that all of these coincidences are not coincidences at all, but the result of very conscious human knowledge.
Let us take a maya number/fractal (the alautun 2304 number) and carry out the same exercise.
| 360 - 230.4 = 129.6 (kemi) | 378 - 230.4 = 147.6 (maya apparent) |
Or, let us take the more obtuse number/fractal of 374.4 (double the maya fractal number 1872):
And, now we can see how 147.6 unites the remainders 75.6 + 72 = 147.6, leading us to believe that all of these numbers coming from two distinct reckoning systems are intimately related. It is simply a question of discerning how they might be related. The numbers pertaining to the radian and the anti-radian listed on the previous table of functions may be one of the tools in comprehending why certain numbers/fractals were chosen for the computations. Let us explore the numbers in relation, then, to the theoretical analysis of the features of a circle.
Other authors, like Carl P. Munck and Hugh H. Harleston, Jr., have pointed out the significance of the radian in analyzing the ancient reckoning system and artwork. We became somewhat convinced of the significance of the radian when we observed its relationship to the number/fractal encoded into the Sothic cycle, 1649.457812, which we discussed previously (Cfr., Earth/matriX Essay No. 73). The 1649.457812 number/fractal is apparently without significance; unlike any number/fractal encountered in the ancient reckoning system. Yet, observe its apparent relationship to the radian.
| 1649.457812 x 57.29577951 | = | 9450.697111 18901.39422 37802.78844 75602.78844 151211.1538 302422.3075 (ca. anti-radian) |
With such precise coincidence of numbers, we elaborated the table of functions of the radian and the anti-radian in order see the relationship of the opposing angles for the entire 360-degree circle. On the table one may observe specific patterns.
In the ancient reckoning systems of Mesoamerica there are two calendars of primary significance, the one based on the 260c day-count and the one based on the 360c day-count. The 260c reflects a relationship of 13 months consisting of twenty days each. While the 360c consists of 18 months and twenty days each. In this sense, the 13c and the 18c are significant. Let us review these counts on the table of radians.
***
The 13c and 18c on the Radian and Anti-Radian Table of Functions
Reproduction prohibited.
| Home | Books | Forum | Reviews | Links | Author |