A New Special Right Triangle: 15° · 75° · 90°

The .366 | 1.366 | 2 Baseline of the
Special Right Triangle Series

15° · 75° · 90°

30° · 60° · 90°

45° · 45° · 90°

Charles William Johnson

Extract

In a previous analysis, Fractal Triangles, I explored a series of triangles that complement the basic and special triangles that are presented in textbooks on geometry. In this essay, I will present a baseline triangle that, in my view, has been overlooked throughout the study of geometry. It is my interpretation that for centuries this series of triangles has had an undue emphasis placed upon the basic and special triangles, as though these were in fact the only relevant triangles.

see version pdf: base_triangle_series.pdf

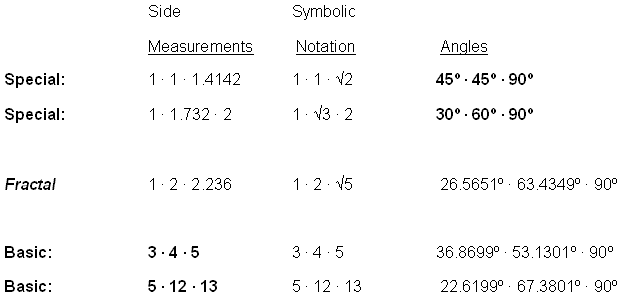
In a previous analysis of a series of fractal triangles, I illustrated how the fractal triangles are complementary to the traditionally cited basic and special triangles. A summary view of the basic and special triangles presented in textbooks on geometry reads as follows.

The basic triangles 3 • 4 • 5 and 5 • 12 • 13 are generally cited in most textbooks on geometry. These triangles are presented as of their side measurements. While the special 45º • 45º • 90º and 30º • 60º • 90º triangles are presented as of the measurements of their angles. The reasons for this arbitrary presentation concern an age-old desire to work with whole numbers. It has been cited that the ancients avoided the decimal expressions of irrational numbers; and this appears to be still the case today. Although treatments of the triangles 1 • 1 • 2 and 1 • 3 • 2 are common, hardly ever does one find any elementary study related to the triangle 1 •2 • 5. Again, this may have to do with the fractional expression of its angles. The angles of the 1 • 2 • 5 triangle are: 26.5651º • 63.4349º • 90º. Small wonder this triangle is referred to by its side measurements albeit symbolically by the square root of 5. 1 • 2 • 5 which translate into the side measurements of 1 • 2 • 2.236067978.

The two different series of right triangles, along with a fractal triangle, then, may be summarized in the following manner:

As I gaze upon this particular placement of the different triangle series, one element stands out: the logic of considering a triangle of 15º • 75º • 90º. In other words, logic would tell us that one might consider a series of triangles as of increments/decrements of fifteen degrees:

45º • 45º • 90º

30º • 60º • 90º

15º • 75º • 90º

One can only wonder why the series of special triangles has not been presented in its obvious progression, and only two of the triangles within the series have been emphasized in textbooks on geometry over the centuries. In order to understand the possible significance of considering the 15º • 75º • 90º special triangle, along with its corresponding series a few comments are in order regarding the Earth/matrix temperature scale.

For the past few years, I have been presenting the idea of utilizing a thermodynamic temperature scale that has the boiling point of water (BPW) as unit one (1.0) and/or the freezing point of water (FPW) as unit one (1.0). Variations of these scales have been presented in the Earth/matriX series of essays, but I will concentrate on the scale that employs the freezing point of water as unit one in this essay. If one assigns the freezing point of water as unit one (1.0), then the boiling point of water will be registered as 1.3661 on that same scale, where absolute zero is precisely 0.00. The difference, then, between the freezing point of water (1.0) and the boiling point of water (1.3661) is represented in the value of .3661 on the Earth/matriX thermodynamic temperature scale.......

see version pdf: base_triangle_series.pdf

©2007-2014 Copyrighted by Charles William Johnson. All rights reserved.Earth/matriX:
Science in Ancient Artwork. johnson@earthmatrix.com